The quadratic equation is a polynomial of second degree with the following formula: f(x) = ax2 + bx + c where a, b, c, ∈ R, and a ≠ 0. A leading coefficient is referred to as ‘a’, while ‘c’ is the absolute term of f (x).
The unknown variable in a quadratic equation has two values, usually referred to as the roots (α, β). Factoring an equation allows us to obtain the roots of a quadratic equation.
Understanding of Perfect Square Trinomial:
A fundamental skill for solving algebraic expressions that involve polynomials is the ability to recognize special cases of polynomials that can easily be factored in.Trinomials of the perfect square type are among these “easy to factor”polynomials. The term trinomial refers to an algebraic expression composed of three terms that are added or subtracted together.
An expression composed of two terms is called a binomial. Therefore, a perfect square trinomial is a squaring of a binomial expression. A perfect square trinomial can be factored after learning how to recognize it.
A perfect square trinomial can be identified using the following tips:
- Make sure that the first and last terms of the trinomial are perfect squares.
- You can do this by multiplying the roots of the first and third terms.
- In step two, compare the middle term result with that of the first term.
- The expression is a perfect square trinomial if the first and last terms are perfect squares, and the coefficient of the middle term equals the product of the square roots of the first and last terms.
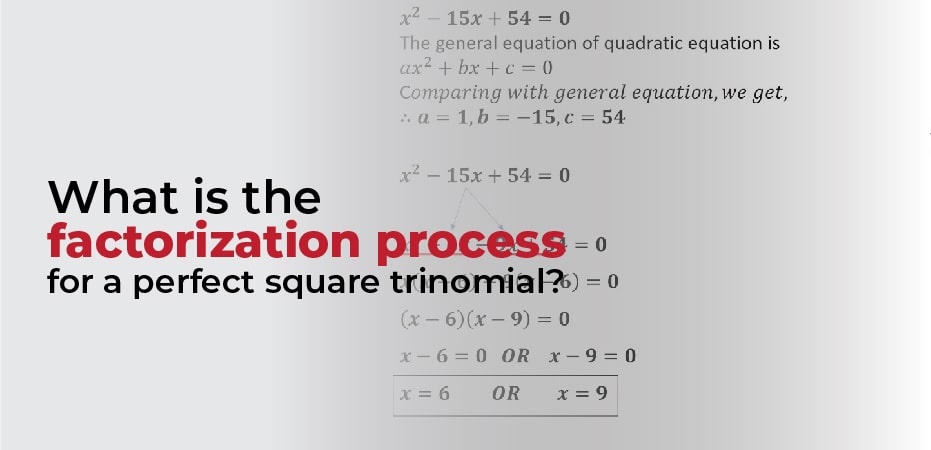
What is the factorization process for a perfect square trinomial?
The process of factoring a perfect square trinomial is quite straightforward when you can locate a perfect square.It is important to understand the steps involved in factoring a perfect square trinomial.
- The squared numbers in the first and third terms of the trinomial should be identified.
- If there are negative or positive numbers in the middle term, it has to be identified. In the case of a positive or negative middle term of the trinomial, the factors will be indicated by a plus and a minus sign, respectively.
- In order to apply the identities below, you will need to write the following terms
- a2 + 2ab + b2 = (a + b)2 = (a + b) (a + b)
- a2 – 2ab + b2 = (a – b)2 = (a – b) (a – b)
Perfect Square Formula – Trinomial Algebra:
A perfect square trinomial can be written as an expression obtained from the square of a binomial equation. In order for an expression to be a perfect square trinomial, it must have the following form ax2 + bx + c and satisfy the condition b2 = 4ac.
A perfect square trinomial expression is written in the following way:
- (ax)2 + 2abx + b2 = (ax + b)2
- (ax)2 −2abx + b2 = (ax−b)2
- Example No 1:
Factor x2+ 6x + 9
Solution:
We can rewrite the expression x2 + 6x + 9 in the form a2 + 2ab + b2 as;
X2+ 6x + 9 ⟹ (x)2 + 2 (x) (3) + (3)
Applying the formula of a2 + 2ab + b2 = (a + b)2 to the expression gives;
= (x + 3)2
= (x + 3) (x + 3)
- Example No 2:
Factor x2 + 8x + 16
Solution:
Write the expression x2 + 8x + 16 as a2 + 2ab + b2
X2 + 8x + 16 ⟹ (x)2 + 2 (x) (4) + (4)2
Now we will apply the perfect square trinomial formula;
= (x + 4)2
= (x + 4) (x + 4)
